How Fast Do Spacecraft Travel in The Expanse - Wired
OK, so we have a problem (yes, I know this is a science fiction show and not meant to be analyzed). Is the acceleration displayed incorrectly? Is the speed incorrect? Maybe the units for speed aren't meters per second? In order to proceed, I want to keep the acceleration at 3.15 g's—that means I'm going to have to fix the speed. The simplest way is to call the "M" in MPS something other than meters. Let me start by finding the conversion between meters and M (whatever that stands for). I can set the two accelerations equal to each other and solve for M.
I will call M the Martian-meter. It's shorter than an Earth-meter. Oh wait! What if the acceleration is not 3.15 Earth-g's but 3.15 Martian g's? The gravitational field on the surface of Mars is 3.71 N/kg (3.71 m/s2) which would mean that 3.15 g's would be an acceleration of 11.7 m/s2. That's not good. That makes the acceleration in the clip in greater disagreement with the change in velocity. OK, I'm going with the Martian-meter idea (and I'm sticking to that).
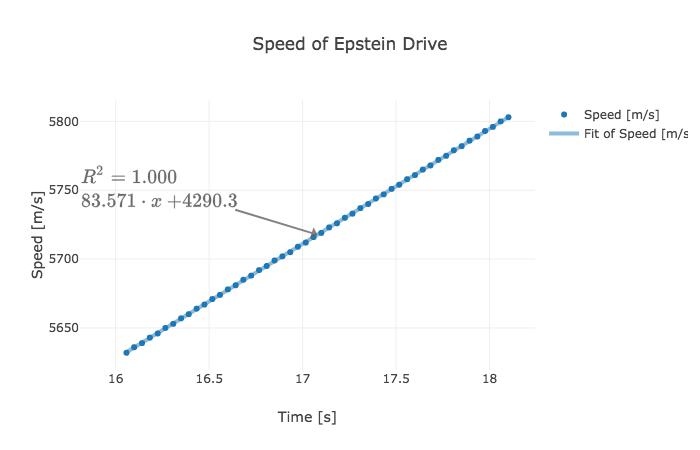
The next time the scene shows the control panel is at a "run time" of 2 minutes and 12 seconds.The acceleration is listed at 4.28 g's. If I record the rate the speed changes again, it's very linear with an acceleration of 617.07 M/s2 (notice that I am using Martian-meters) or 228.3 m/s2 (Earth-meters). Converting the acceleration on the panel, I get 4.28 g's equal to 41.94 m/s2. OK, here is a news flash. I don't think the numbers really mean anything except that they are increasing at a linear rate.
Now for a comment. As someone who consults shows regarding science content, I suspect I know how this happened. Some science person calculated the speed so that it agrees with the 4.28 g acceleration. Next the special effects people made a program that displays the calculated speed on the readout in the scene. Finally, a producer or director looked at the rough cut and said "Hey, that doesn't look very fast. Can we make the speed change even more?" Boom, the display is different. And really, I'm OK with this—they are trying to tell a story and emphasize the huge acceleration. Who would really check that stuff anyway? Oh, that's right—me.
But wait! It gets even worse. If you measure the acceleration based on the changing speed, it gets high—very high. At the end of the clip, the spacecraft is traveling around 25 million meters per second and has an acceleration of about 46,119 m/s2. That's the equivalent of 4,700 g's. Boom.
-
Latest
 What is the fastest particle in the universe?
What is the fastest particle in the universe?What is the fastest particle in the universe?tachyonWhat is the smallest thing in our galaxy?In a cosmic version of the old needle-in-a-haystack finding, astronomers have spotted an object less than a...
-
Next
 Question: What Is Fastest Thing In The Universe ...
Question: What Is Fastest Thing In The Universe ...Has any car hit 1000 mph?A new vehicle from the Bloodhound Project, called the SuperSonic Car (SSC), is built to surpass speeds of 1,000 miles per hour, according to CNN.In 2016, the makers plan to b...
Popular Articles
- Rocket Power (TV Series 1999–2004) - Rocket Power (TV Series ...
- technique - What is the definition of 'playing in the pocket ...
- "Pocket rockets," in poker Crossword Clue Answers, Crossword ...
- 5 Sex Toys Every Man Should Own, Use & Use Again - LA Weekly
- Pocket Holsters: 11 Options For Easy Everyday Carry (2021 ...
- What is Elton John's most successful song? (Celebrity Exclusive)
